Life Skills: Finance Understanding interest

If you have ever looked at a savings account, or a loan, or buying an item on finance, you may have heard of interest, compound interest, and APR. 

We will go over interest and explain the difference between compound interest and simple interest. We have an extract from a book provided by Young Enterprise Scotland, and the link to download the free PDF will be in the references section.

Compound vs. Simple interest

Let's start off by looking at the two different interest types and how they work: we found these handy pages that accurately and relatively easily outline how interest works.

There are two ways of calculating interest – simple interest and compound interest. In reality, all banks will use the compound method to work out the interest they pay or charge you, but let’s look at the simple interest method first to see why this is:

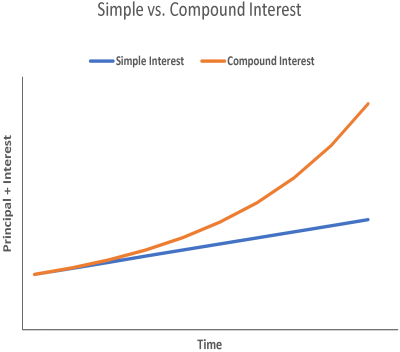
Simple Interest 

The interest earned on an initial amount of  £1,000 (known as the Principal) deposited into a savings account with an interest rate of 2%, or 0.02  in decimal format (known as the Rate), for 1 year (known as the Time) can be worked out using the simple interest formula:

Interest = P x R x T

In this case, this would be 1,000 x 0.02 x 1. 
So, an interest rate of 2% paid on a principal of £1,000 would gain £20 interest over 1 year. This will only be accurate if no further money, on top of the original £1,000, is deposited or withdrawn. This method of calculating interest is known as the simple interest method. It suggests that the principal amount would earn £20 every year.

In reality, organizations use a different method of calculating interest known as compound interest. This recognizes that at the end of year 1, your total savings would be £1,020 and that in year 2 you should have your interest calculated against this higher amount. This means that the interest gained in previous years will also earn interest – meaning you receive a higher return on top of your savings.  

Compound Interest, or interest upon interest upon interest…

So, if the £1,000 is deposited for 3 years and the 2% rate is compounded it will work out as follows:
Year 1: £1,000 x 0.02 x 1 = £20.00 interest. Total savings at the end of Year 1 = £1,020
Year 2: £1,020 x 0.02 x 1  = £20.40 interest. Total savings at the end of Year 2 = £1,040.40
Year 3: £1,040.40 x 0.02 x 1  = 20.81 interest. Total savings at the end of Year 3 = £1,061.21    

So, over 3 years with a principal sum of £1,000 you would receive £1.21 more if the interest was calculated using the compound method compared to the simple interest method. Not a huge difference? Well, that’s true on smaller amounts saved over short periods but, for larger amounts over longer time periods, the interest gained will be much more significant. This method of calculating compound interest is really good to use when there are only a small number of years to calculate but imagine if you had savings of 10 years or more… it would take you much longer to work out the answer. Therefore, another way of calculating compound interest is using the multiplier, for example, if £50,000 was saved over 15 years at an interest rate of 2% the total value of savings would be: 
£50,000 x (1.02)^15=£67,293

So, the amount of interest earned over 15 years would be:
£67,293 - £50,000 = £17,293

If we had used the simple interest method, the total interest would have been:
£50,000 x 0.02 x 15 = £15,000, so the total value of the savings would have been £65,000.

In this scenario, using the compound method would mean you would receive £2,293 more interest.  That’s £2,293 more, just because of the way the interest is calculated. This final amount will only be reached if no further money is deposited or withdrawn. In reality, more money may have been added to the amount being saved or some of it taken out to be spent. Therefore, the amount actually being saved will vary constantly; this will, in turn, affect the amount of interest being accrued.

Extracted from the Young Enterprise - Your Money Matters Financial Education Textbook 

Why is this useful?

Knowing how your interest is calculated is hugely important for instance if you are looking for savings account for saving up a large sum of money you will want to make sure you take out a savings account with a compound interest rate.

Also if you take out a mortgage most mortgages work on a compound interest so it pays to try to make extra payments to reduce the interest charged on your mortgage.

Interest by other names

You might be familiar with the terms APR, or AER, but we’re going to give you a quick breakdown of these terms are, where you can find them and what they mean.

To make it simple it is best to think of anything that says APR is usually a loan or form of credit you are taking out and is used to calculate the amount of interest you will have to pay. Whereas AER is often used to refer to the interest in your accounts mainly bank accounts like savings accounts and is used to calculate the interest that will be paid to you. So let us get into them a bit more:

APR

APR stands for the Annual Percentage Rate, and it's the official rate used for borrowing. When it's calculated  it has to include:

• The cost of the borrowing, so the amount of interest charged plus
• Any associated fees that are automatically included, such as an application or annual fee

An APR is therefore meant to give you the overall equivalent cost of a debt, which you can then use to compare against other credit and loan products. It must be displayed by a lender before any agreement is signed. 
The fact it includes charges sometimes means the APR can be a bit confusing. For example, an interest rate could be 22.2% per annum but the APR is 27.3%, as the impact of a £25 annual fee adds the equivalent to an-other 5.1% interest. Yet this is useful as it allows a true comparison.

AER

The AER, or Annual Equivalent Rate, is the official rate for savings accounts and is designed to allow you easily compare the differences between savings accounts. (it's the equivalent of the APR for debts). But when you really get into it, it can become a little more complex so below we have paraphrased information from the following websites and money advice services: Moneysaving expert, Money advice services, Money supermarket the links to these pages will be in the reference section.

Here is the definition from Money Advice Service:

Annual Equivalent Rate (AER) – this is the total interest you’ll receive on your savings in one year. Shown as a percentage, the higher the AER, the more interest you’ll receive.
AER is a little more complex than APR in the sense that when looking at savings accounts and interest rates in your bank you may come across a Gross Rate, so here is a brief explanation of the two that has been paraphrased from money supermarket:

The Annual Equivalent Rate is the official rate of savings accounts and makes it easier to compare rates offered by different banks and building societies. The AER Calculates your interest rate based on if you were to leave your money in the savings account for a year without withdrawing any money from it, but it also factors in the compound interest.

As a result, when you look at the monthly interest paid by an account the AER shown will be higher than the gross rate. This is to make sure the AER is not also calculating from the gross interest. However, where interest is paid annually, the gross rate and the AER will be the same.

The idea is it shows what you'd get over a year if you put money in the account and left it there. The alternative is the gross rate, which is the flat rate of interest that's actually paid.

The gross interest rate is the amount of interest you will earn before income tax is deducted and like the gross rate, the AER shows how much interest you will earn before tax is deducted.

The main thing you need to remember when you are looking at interest rates for a savings account is to compare the AER of accounts as this is the figure that you will be using to figure out how much you would make in interest on your saving deposit.

Flat Interest Rates

Finally, it is worth mentioning that there is the Third kind of interest that is only used when taking credit, it is known as the Flat Interest Rate. Now it may sound simple enough and if you are taking something out on finance or credit the seller may make it sound cheaper than an APR rate but don't be fooled. A Flat interest rate is calculated on the total amount of credit you are taking and will stay the same for the remainder of the agreement. so for example, if you buy a car for £10,000 for 10 years and the flat interest rate was 5% that would mean you would be paying £500 every year till you paid off the entire balance. In essence, you may end up paying more interest than you would on an APR.

Reference pages:

Interest and how to calculated them extracted from

https://www.young-enterprise.org.uk/resources/your-money-matters-financial-education-textbook/ 
https://www.thinkmoney.co.uk/blog/whats-the-difference-between-gross-and-net-savings-interest/

AER + APR and gross rates

https://www.moneysavingexpert.com/banking/interest-rates/
https://www.moneysupermarket.com/savings/understanding-interest-guide/
https://www.moneyadviceservice.org.uk/en

Financial Services:

Money Advice Service

National Debtline

Citizens Advice Service

StepChange Debt Charity